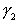Coefficient of Kurtosis

Kurtosis is a measure of the sharpness of the data peak. Traditionally the value of this coefficient is compared to a value of 0.0, which is the coefficient of kurtosis for a normal distribution (i.e. the bell-shaped curve). A value greater than 0 indicates a peaked distribution and a value less than 0 indicates a flat distribution. Without a very large sample size, the use of this coefficient is of questionable value.

Population Kurtosis (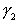 $image\kurt-y2.gif$ )

$image\popkurt.gif$

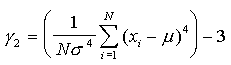
Sample Kurtosis (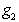 $image\kurt-g2.gif$ )

$image\samkurt.gif$

$image\kurtosis.gif$

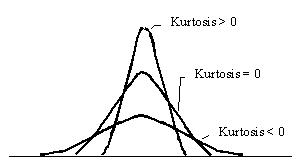
(adapted from King and Julstrom, 1982)

where

$image\popsd-sym.gif$	= Population Standard Deviation
S	= Sample Standard Deviation
$image\u.gif$	= Population Mean
$image\mean.gif$	= Sample Mean
N	= number of data values for a population
n	= number of data values for a sample
xi	= ith data value